Puzzle Solutions
Tower of Hanoi – Inverted Solution:
If the tower consisted of only 1 disk, the puzzle could be completed in only 1 move. If the tower consisted of 2 disks, the puzzle could be completed in 3 moves. if the tower consisted of 3 disks, the puzzle could be completed in 7 moves. For each of these cases, the number of moves is 2n-1. Thus for the 5 disk model, the puzzle could be completed in 25-1=31 moves.
The Travelling Salesman Solution:
The distances between the various cities, as measured in centimeters on the model, are shown on the table below.
| Olympia | Salem | Boise | Helena | Sacramento | Carson City | Salt Lake City | Cheyenne | Denver | Santa Fe | Phoenix | |
| Olympia | 14.1 | 29.8 | 42.2 | 51.2 | 48.3 | 60.2 | 85.1 | 90.4 | 105 | 98.3 | |
| Salem | 14.1 | 29.5 | 49.1 | 38.1 | 36.9 | 56.8 | 86.2 | 90.3 | 101.5 | 89.7 | |
| Boise | 29.8 | 29.5 | 24.9 | 41.6 | 34 | 30.5 | 56.7 | 61.1 | 75.1 | 72.2 | |
| Helena | 42.2 | 49.1 | 24.9 | 66.5 | 58.4 | 39.6 | 47.8 | 55 | 76.8 | 85.8 | |
| Sacramento | 51.2 | 38.1 | 41.6 | 66.5 | 9.3 | 46.9 | 82.4 | 82.7 | 82.9 | 59.1 | |
| Carson City | 48.3 | 36.9 | 34 | 58.4 | 9.3 | 37.7 | 73.1 | 73.6 | 75.2 | 54.7 | |
| Salt Lake City | 60.2 | 56.8 | 30.5 | 39.6 | 46.9 | 37.7 | 35.3 | 36.2 | 44.7 | 46.2 | |
| Cheyenne | 85.1 | 86.2 | 56.7 | 47.8 | 82.4 | 73.1 | 35.3 | 8.7 | 36.2 | 64 | |
| Denver | 90.4 | 90.3 | 61.1 | 55 | 82.7 | 73.6 | 36.2 | 8.7 | 27.5 | 57.9 | |
| Santa Fe | 105 | 101.5 | 75.1 | 76.8 | 82.9 | 75.2 | 44.7 | 36.2 | 27.5 | 38.8 | |
| Phoenix | 98.3 | 89.7 | 72.2 | 85.8 | 59.1 | 54.7 | 46.2 | 64 | 57.9 | 38.8 |
Selecting Salt Lake City as the starting city, a Mathematica computer program ran for 2 days to arrive at the path that generated the shortest distance of 276.7 cm. That path is
Salt Lake City – Carson City – Sacramento – Salem – Olympia – Boise – Helena – Cheyenne – Denver – Santa Fe – Phoenix
The path from Salt Lake City with the second shortest length of 281.2 cm is
Salt Lake City – Cheyenne – Denver – Santa Fe – Phoenix – Carson City – Sacramento – Salem – Olympia – Boise – Helena
If the starting city is Boise, the shortest path of 276.6 cm is
Boise – Helena – Olympia – Salem – Sacramento – Carson City – Salt Lake City – Cheyenne – Denver – Santa Fe – Phoenix
The path from Boise with the second shortest length of 284.6 cm is
Boise – Helena – Olympia – Salem – Carson City – Sacramento – Salt Lake City – Cheyenne – Denver – Santa Fe – Phoenix
The Which is Fastest Solution:
The How Far Out Solution:
Please click here for a pdf of the solution.
The Penrose Puzzle Solution:
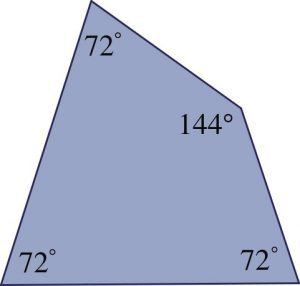
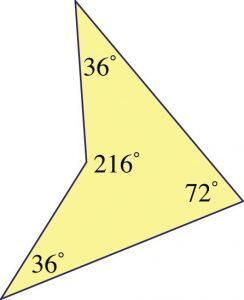
An interesting property of the blue kite and yellow dart is the measures of their interior angles. It turns out that 144 degrees is the measure of an interior angle of a regular decagon while 36 degrees is the measure of the exterior angle of a regular decagon. The central angle and exterior angle of a regular pentagon measures 72 degrees. These angle properties lead to symmetries in the tessellations you create similar to those found in a regular pentagon or regular decagon. For example there are many places in the Penrose tilings where 5 darts or 5 kites come together because 5 angles of 72 degrees is 360 degrees.

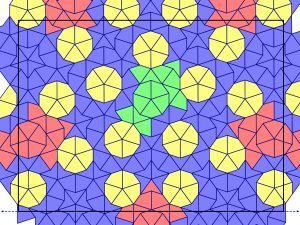
In the figure above, notice the decagons outlined in green and the five red lines of symmetry. As mentioned in the information on the table, the angles of the kite and dart are related to the angles of a regular pentagon and regular decagon which contributes to the appearance of the properties of these polygons in the tessellations.
Although the tiling below is different from the one above, you will still notice many symmetries related to the regular pentagon and regular decagon.

Find the Bears Solution:
The image below displays six bears and another six bears can be found in those same locations by rotating the current bears 180 degrees. Thus the total number of bears is 12. If, however, you do not count the orange bear at the right as being completely within the rectangular boundary then there are only 10.

Find the Fish Solution:
An interesting property of the kite (in our tiling some are blue and some are yellow) and the dart (in our tiling all of the darts are blue) is the measures of their interior angles. It turns out that 144 degrees is the measure of an interior angle of a regular decagon while 36 degrees is the measure of the exterior angle of a regular decagon. The central angle and exterior angle of a regular pentagon measures 72 degrees. These angle properties lead to symmetries in our tiling similar to those found in a regular pentagon or regular decagon. For example, there are many places in our tiling where five darts or five kites come together because five angles of 72 degrees is 360 degrees.


In our tiling, there are five fish similar to the green one in the image below all around that center shape of five kites forming a decagon. You will notice that all polygons immediately adjacent to this decagon are darts. There are no other complete fish on the tiling within the rectangle boundary because there are no other decagons that are completely surrounded by darts. If, however, we extended the boundary, there are several other locations similar to the one in the center where the decagon is surrounded by darts. You can see the possible fish in red in each of these locations. Since none of these red fish are within the rectangle boundary, the total number of fish in our tiling is five!
Tangram:
The solutions for the Tangram table are sown in the figure below.
